Leaving flatland – quantum Hall physics in 4-D

In physics, on the other hand, the possibility that our universe comprises more than three spatial dimensions was first proposed in the wake of Albert Einstein’s theory of general relativity in the 1920s. Modern string theory – trying to reconcile Einstein’s ideas with the laws of quantum mechanics – even postulates up to 10 dimensions.

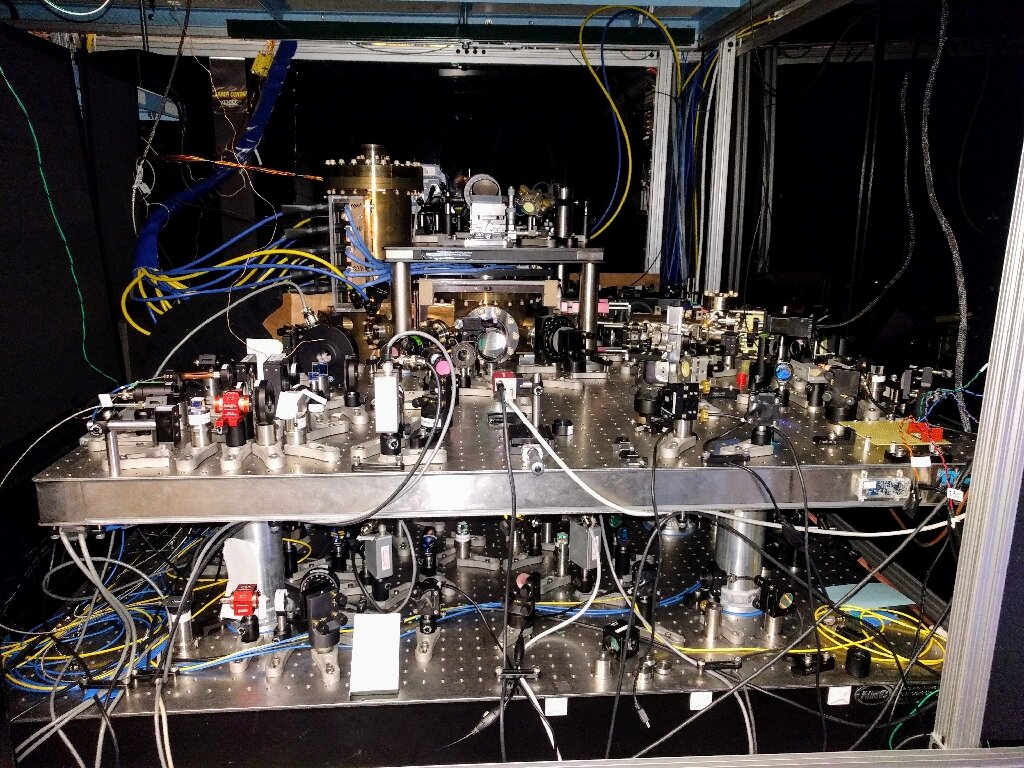
In a completely different context, an international team of researchers led by Professor Immanuel Bloch (LMU/MPQ) and Professor Oded Zilberberg (ETH Zürich) has now demonstrated a way to observe physical phenomena proposed to exist in higher-dimensional systems in analogous real-world experiments. Using ultracold atoms trapped in a periodically modulated two-dimensional superlattice potential, the scientists could observe a dynamical version of a novel type of quantum Hall effect that is predicted to occur in four-dimensional systems.

The Hall effect occurs when charged particles move in a two-dimensional plane in the presence of a magnetic field. The magnetic field generates a Lorentz force, which deflects the particles in the direction orthogonal to their motion. This manifests in the appearance of a transverse Hall voltage. In 1980, Klaus von Klitzing made the remarkable discovery that at low temperatures and very strong magnetic fields this voltage can only take certain quantized values.

Moreover, these values are identical irrespective of the specific properties of the experimental sample. This astonishing fact was later shown to be related to the topology of the quantum mechanical wave functions describing the behaviour of electrons at such low energies – a seminal work for which David Thouless was awarded the Nobel prize in physics in 2016.

An important prerequisite for the quantum Hall effect turned out to be the two-dimensional geometry of the sample. It can be proven that in general such a phenomenon cannot take place in three-dimensional systems – as exemplified by the fact that the direction transverse to the velocity of the particles is not defined uniquely in three dimensions. Thus, it was believed that this effect is special to two dimensions.

Yet, 20 years after the initial discovery theoretical physicists postulated that a similar effect could also take place in four-dimensional systems, for which even more remarkable properties including a novel non-linear Hall current were predicted. For a long time, however, this proposal was mostly regarded as a mathematical curiosity – out of reach for actual experiments – despite its far-reaching implications. For example, both topological insulators and Weyl semimetals, two of the most prominent discoveries in condensed matter physics in recent years, can be derived from 4-D quantum Hall models.

Read more at: https://phys.org/news/2018-01-flatland-quantum-hall-physics-d.html#jCp